Last update=20 May, 2006

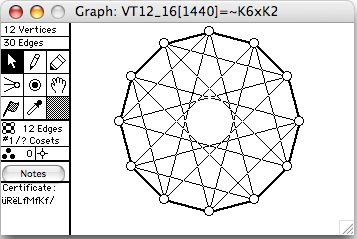
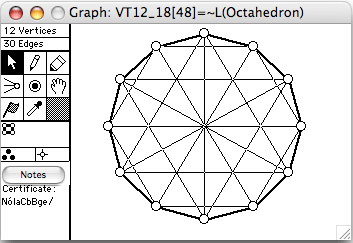
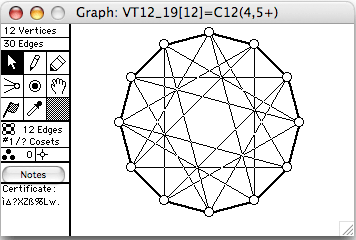
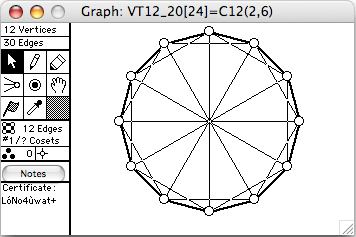
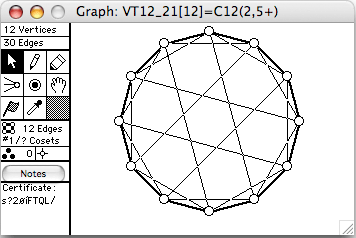
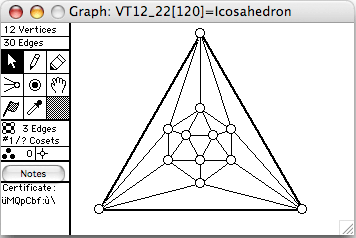
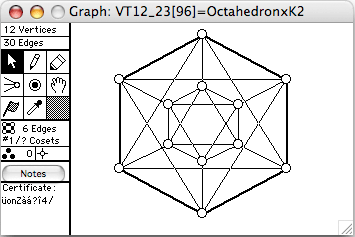
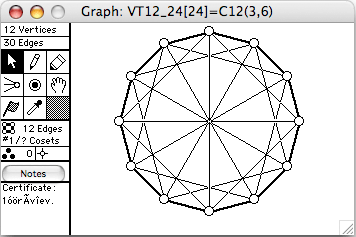
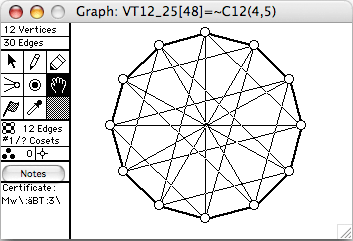
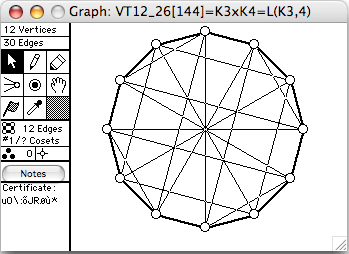
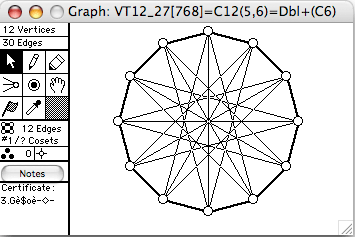
There are 64 connected vertex-transitive graphs on 12 vertices. The 12 of degree 5 (hence 30 edges) are shown here. The order of the automorphism group is given in square brackets in each window's title.
Notation:
- Cn means the cycle of length n
- Cn+ means the cycle of length n with diagonals
- Cn(k) means the cycle of length n with chords of length k
- Cn(k+) means the cycle of length n with chords of length k from every second vertex
- ~G means the complement of G
- 2G means two disjoint copies of G
- GxH means the direct product of G and H
- Prism(m) means CmxK2, ie, two cycles with corresponding vertices joined by a matching
- trunc(G), where G is planar, means to truncate G, ie, replace each vertex of degree k by Ck
- L(G) means the line-graph of G
- Octahedron means the graph of the octahedron; this is L(K4) or ~3K2 or C6(2)
- Icosahedron means the graph of the icosahedron
- Dbl(G) means the double of G. Make 2 copies of G, call them G1 and G2. If uv is an edge of G, then u1v2 and v1u2 are also edges of Dbl(G)
- Dbl+(G) means the double of G, with the additional edges u1u2
- antip(G) means the antipodal graph of G. It has the same vertices, but u and v are joined in antip(G) if they are are maximum distance in G
The complements of the graphs shown here are:
- VT12_28 = ~2K6=Dbl(K3,3)
- VT12_29 = ~(K3xK4)
- VT12_30 = ~Icosahedron
- VT12_31 = C12(3,4)=~C12(2,6)
- VT12_32 = ~C12(5,6)=Dbl(Prism(3))
- VT12_33 = C12(2,4)=~C12(3,6)
- VT12_34 = L(Octahedron)=L(L(K4))
- VT12_35 = ~C12(2,5+)
- VT12_36 = C12(4,5)
- VT12_37 = ~C12(4,5+)
- VT12_38 = ~(OctahedronxK2)
- VT12_39 = C12(2,3)=~C12(4,6)
- VT12_40 = K6xK2=L(K2,6)
- VT12_29 = ~(K3xK4)
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |

 Back to the Groups & Graphs home page.
Back to the Groups & Graphs home page.